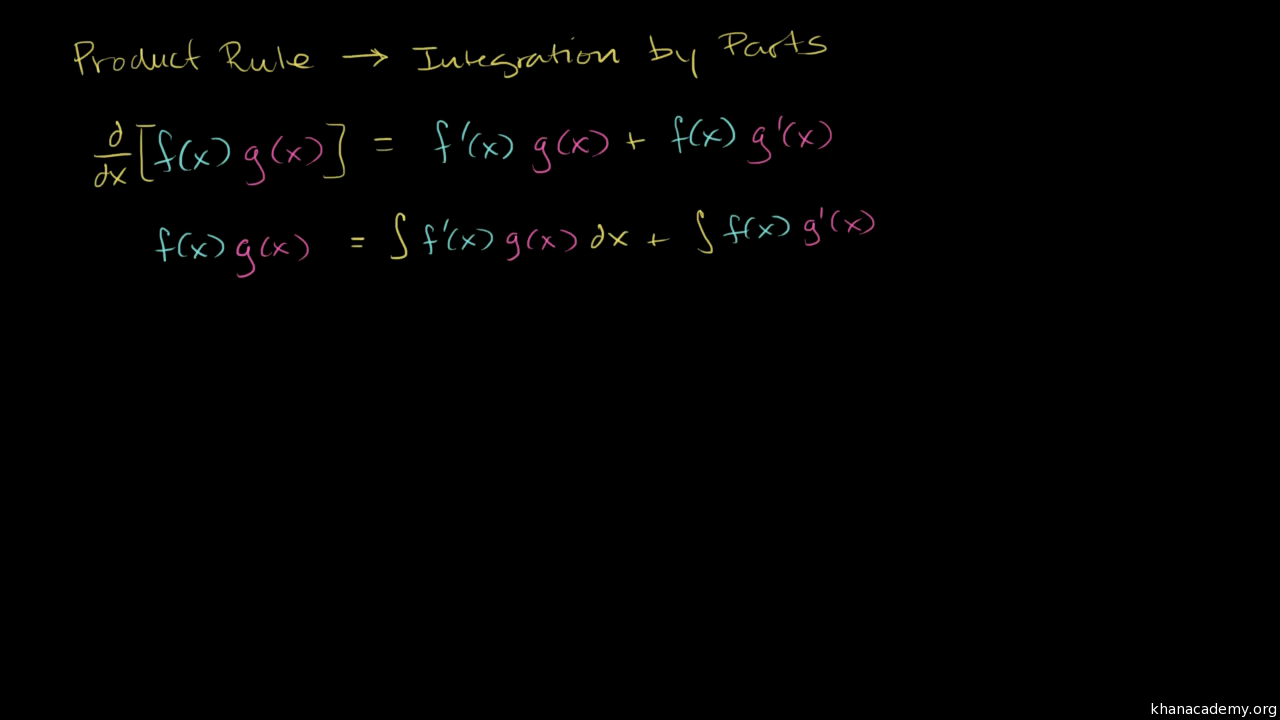Spectacular Khan Academy Reduction Formula

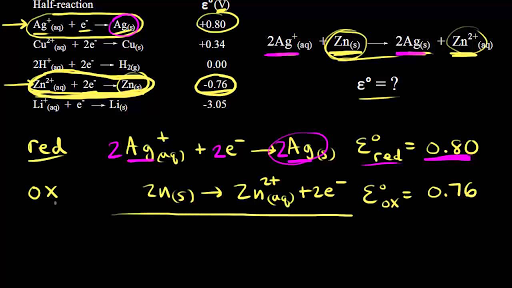
Reduction formula is regarded as a method of integration.

Khan academy reduction formula. The procedure however is not the same for every function. Sin21 cos 2 19 These are sometimes called power reduction formulas because they allow us reduce the power on oneof the trig functions when the power is an even integer. Learn for free about math art computer programming economics physics chemistry biology medicine finance history and more.

Using instructional videos the Khan Academy website provides additional explanations of concepts in the Math Review for the Quantitative Reasoning Measure of the GRE General Test PDF. This file will unzip into a folder named 84 Chemistry Videos by Khan Academy. By looking at the product rule for derivatives in reverse we get a powerful integration tool.

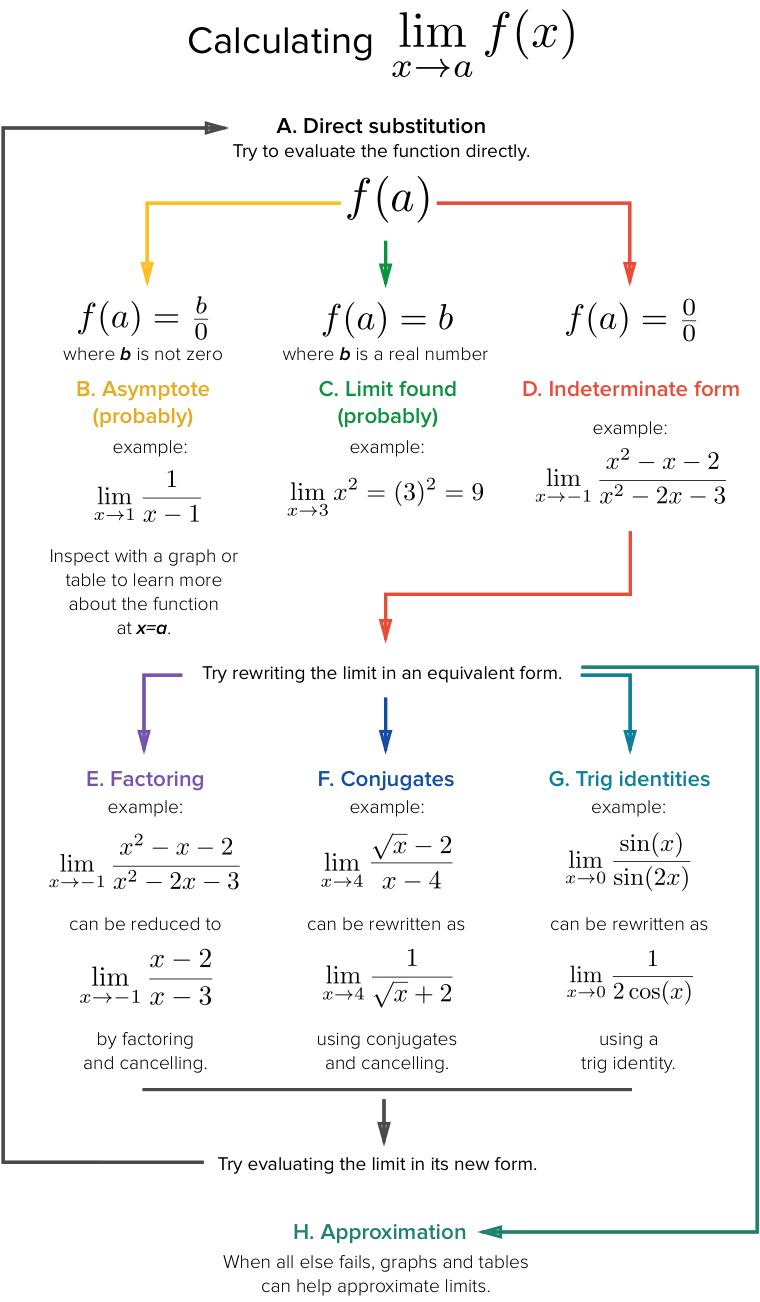
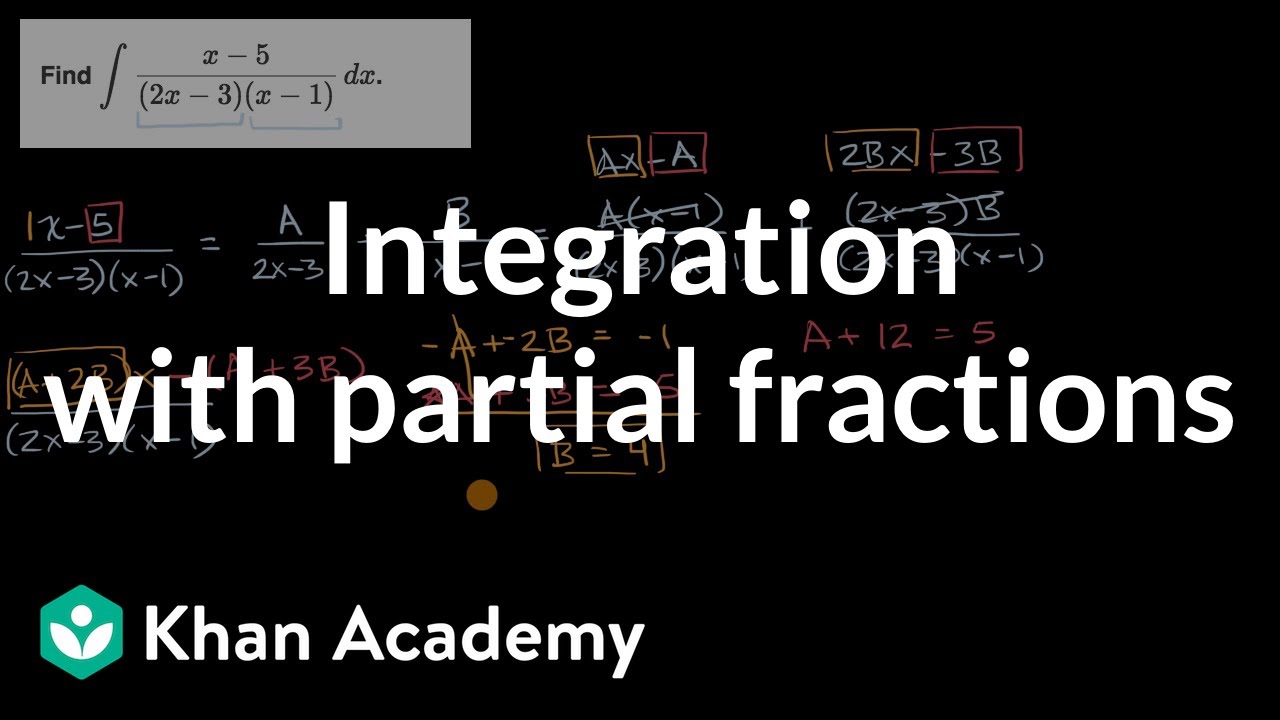
The reduction formula can be derived using any of the common methods of integration like integration by substitution integration by parts integration by trigonometric substitution integration by partial fractions etcThe main idea is to express an integral involving an integer parameter eg. The reduction formulae can be extended to a range of functions. To find some integrals we can use the reduction formulas.

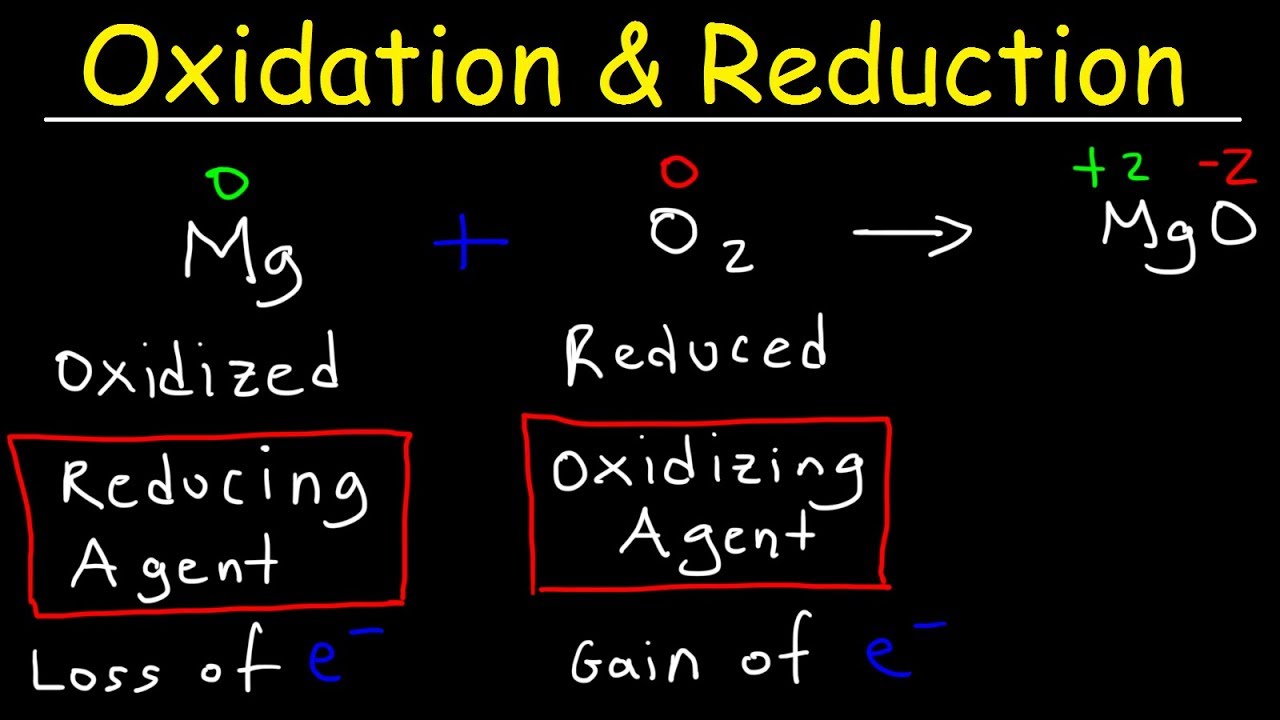
According to the redox rules Ive learned the reaction should be 2H2O 2H2O I2 2H2O2 2I 4OH-. These formulas enable us to reduce the degree of the integrand and calculate the integrals in a finite number of steps. Below are the reduction formulas for integrals involving the most common functions.

In this video we work through the derivation of the reduction formula for the integral of cosnx or cosxnThe first step is to rewrite the integral a. Created by Sal KhanPractice this lesson yourself on KhanAcade. For each topic in the Math Review the table below provides links to relevant sections on the Khan Academy website that contain instructional videos on the topic.

Khan Academy is a nonprofit with the mission of providing a free world-class education for anyone anywhere. What were going to do in this video is review the product rule that you probably learned a while ago and from that were going to derive the formula for integration by parts which could really be viewed as the inverse product rule integration by parts so lets say that I start with some function that can be expressed as the product f of X it can be expressed as a product of two other. 84 Chemistry Videos by Khan Academyzip Here are 84 Chemistry videos from Khan Academy teaching and demonstrating chemistry for a first-year high school or college course.